Summary

---

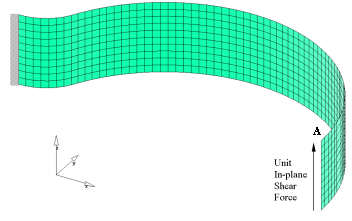
Raasch challenge is a curved strip hook problem with a tip in-plane shear load, posed in 1990 by Ingo Raasch of BMW in Germany. The problem poses a significant challenge to shell elements because of the inherent coupling between three modes of deformation: bending, extension, and twist. OptiStruct is benchmarked against the Raasch challenge to assure its shell elements performance on linear static analysis.

 

Benchmark Model

Three types of elements are used for linear static analysis of the Raasch's hook. They are tria-shell, quad-shell, and hexa-solid elements. For each type of element, five different mesh densities (1x9, 3x17, 5x34, 10x68, and 20x136) are used to investigate the convergence of the solution. Two elements are used in the thickness direction for solid elements, thus, the mesh densities become 1x9x2, 3x17x2, 5x34x2, 10x68x2, and 20x136x2. The target is the z-directional displacement at point A, the upper end corner of the hook. The target value is 4.9366 inches.

 

Linear Static Analysis Results

All results are normalized with the target value.

 

File Location

<install_directory>/demos/hwsolvers/optistruct/Rhex.fem
<install_directory>/demos/hwsolvers/optistruct/Rquad.fem
<install_directory>/demos/hwsolvers/optistruct/Rtri.fem

 

Reference

Knight, Jr. N. F., Raasch Challenge for Shell Elements, AIAA Journal, Vol. 35, No. 2, February 1997.