Both MF variants are completed with a non-linear first order transient force lagging approach. With the kind of non-linearity used, it is taken into account that the decrease in contact forces might take place faster than a respective increase. This effect introduces a certain frequency-dependency of the mean tire force decrease, if wheel load variations apply.
Mathematically, the non-linear tire lag is described by the following non-linear first order differential equation, used for side force, longitudinal force, and aligning torque:
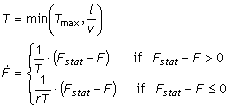
The following parameters and signals are used here:
| • | 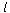 is the constant relaxation length is the constant relaxation length |
| • |  is the time dependent rolling speed is the time dependent rolling speed |
| • | 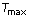 is the constant upper bound for the resulting time constant is the constant upper bound for the resulting time constant |
| • | 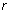 is a reduction factor, describing the relation between time constant for force increase and time constant for force decrease is a reduction factor, describing the relation between time constant for force increase and time constant for force decrease |
| • | 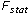  is the stationary value of the respective force or torque (longitudinal force, side force, or aligning torque, respectively) is the stationary value of the respective force or torque (longitudinal force, side force, or aligning torque, respectively) |
| • | 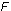 is the resulting transient value of this force or torque is the resulting transient value of this force or torque |
See Also:
Modeling Approach








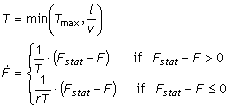
 is the stationary value of the respective force or torque (longitudinal force, side force, or aligning torque, respectively)
is the stationary value of the respective force or torque (longitudinal force, side force, or aligning torque, respectively)