|
»Click here to display Table of Contents«
|
Straight Cantilever Beam |
|
|
|
|
|
Straight Cantilever Beam |
|
|
|
|
|
»Click here to display Table of Contents«
|
Straight Cantilever Beam |
|
|
|
|
|
Straight Cantilever Beam |
|
|
|
|
Length = 6.0; Height = 0.2; Depth = 0.1; E = 1.0E7: Poisson's ratio = 0.3.
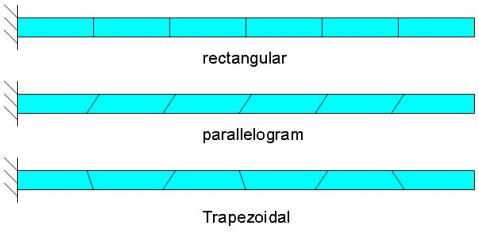
This is a straight cantilever beam solved with solid and shell elements. Three models (rectangular, parallelogram, trapezoidal) are made with each element's type to investigate the effect of distorted elements with a high aspect ratio.
Six types of elements are used for this problem. They are tria-shell, quad-shell, and hexa-solid elements, each with 1st and 2nd order. Four loading cases are used for each model; extension, in-plane bending, transverse bending, and twist. For the extension and bending load cases, unit loads are applied in a consistent fashion over all of the nodes at the tip of the beam. For the twist load cases, a unit moment is applied at the tip.
Theoretical solutions for the deflections at the tip, computed by beam theory, are as follows.
Load Type |
Component |
Value |
extension |
UX |
0.00003 |
in-plane bending |
UZ |
0.1081 |
transverse bending |
UY |
0.4321 |
twist |
ROTX |
0.03208 |
All results are normalized with the target value.
(a) Rectangular |
||||
|---|---|---|---|---|
in-plane extension |
in-plane bending |
Transverse bending |
Twist |
|
QUAD4 |
1.000 |
0.992 |
0.981 |
0.941 |
QUAD8 |
1.006 |
1.000 |
1.016 |
0.953 |
|
||||
TRI3 |
1.000 |
0.032 |
0.973 |
1.072 |
TRI6 |
1.006 |
0.994 |
1.001 |
0.950 |
|
||||
HEX8 |
0.988 |
0.978 |
0.973 |
0.892 |
HEX20 |
1.008 |
0.992 |
0.992 |
0.905 |
|
||||
(b) Parallelogram |
||||
in-plane extension |
in-plane bending |
Transverse bending |
Twist |
|
QUAD4 |
1.000 |
0.712 |
0.981 |
0.905 |
QUAD8 |
1.008 |
0.999 |
1.015 |
0.937 |
|
||||
TRI3 |
1.000 |
0.012 |
0.955 |
0.931 |
TRI6 |
1.005 |
0.962 |
0.995 |
0.982 |
|
||||
HEX8 |
1.012 |
0.624 |
0.529 |
0.820 |
HEX20 |
1.008 |
0.976 |
0.977 |
0.905 |
|
||||
(c) Trapezoidal |
||||
|
in-plane extension |
in-plane bending |
Transverse bending |
Twist |
QUAD4 |
1.000 |
0.173 |
0.964 |
0.869 |
QUAD8 |
1.005 |
0.981 |
1.015 |
0.950 |
|
||||
TRI3 |
1.000 |
0.019 |
0.965 |
1.175 |
TRI6 |
1.006 |
0.972 |
0.999 |
0.947 |
|
||||
HEX8 |
1.010 |
0.047 |
0.030 |
0.563 |
HEX20 |
1.008 |
0.902 |
0.950 |
0.905 |
|
||||
<install_directory>/demos/hwsolvers/optistruct/CBhex.fem
<install_directory>/demos/hwsolvers/optistruct/CBpent1.fem
<install_directory>/demos/hwsolvers/optistruct/CBpen2.fem
<install_directory>/demos/hwsolvers/optistruct/CBquad.fem
<install_directory>/demos/hwsolvers/optistruct/cbquad2.fem
<install_directory>/demos/hwsolvers/optistruct/cbquad4.fem
<install_directory>/demos/hwsolvers/optistruct/CBtri1.fem
<install_directory>/demos/hwsolvers/optistruct/CBtri2.fem
MacNeal, R.H., and Harder, R.L., A Proposed Standard Set of Problems to Test Finite Element Accuracy, Finite Elements in Analysis and Design, 1 (1985) 3-20.