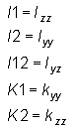Cross Sectional Properties Calculated by HyperBeam

The beam cross section is always defined in a y,z plane. The x-axis is defined along the beam axis. The coordinate system you define is called the local coordinate system; the system parallel to the local coordinate system with the origin in the centroid is called the centroidal coordinate system; the system referring to the principal bending axes is called the principal coordinate system.

For shell sections, only the theory of thin walled bars is used. This means that for the calculation of the moments and product of inertia, terms of higher order of the shell thickness t are neglected. Thickness warping is also neglected.

Area

Area Moments of Inertia


Area Product of Inertia

Radius of Gyration

Elastic Section Modulus


Max Coordinate Extension

Plastic Section Modulus


Torsional Constant	Solid	(see below for warping function)
	Shell open
	Shell closed

Elastic Torsion Modulus	Solid
	Shell open
	Shell closed

Shear Center

Warping Constant (normalized to the shear center)

Shear deformation coefficients



Shear stiffness factors



Shear stiffness
Warping Function	For solid sections, the warping function is computed using a finite element formulation. This may lead to un-physically high stresses in geometric singularities (sharp corners) that get worse with mesh refinement. This may cause problems computing the elastic torsion modulus.