18.2 - Square Membrane Elastic

Title

Square membrane elastic

rad_ex_18.2

Number

18.2 Brief Description

Square plate submitted to two opposing in-plane end forces.

Keywords

•

Q4 shells

•

T3 shells

•	Hourglass, mesh, and concentrated loads

RADIOSS Options

•	Boundary conditions (/BCS)

•	Concentrated loads (/CLOAD)

Input File

4Q4: <install_directory>/demos/hwsolvers/radioss/18_Square_plate/Membrane_elastic/4Q4/.../TRACTION*

8T3: <install_directory>/demos/hwsolvers/radioss/18_Square_plate/Membrane_elastic/8T3/.../TRACTION*

8T3 inv: <install_directory>/demos/hwsolvers/radioss/18_Square_plate/Membrane_elastic/8T3_inv/.../TRACTION*

2Q4-4T3: <install_directory>/demos/hwsolvers/radioss/18_Square_plate/Membrane_elastic/2Q4-4T3/.../TRACTION*

Technical / Theoretical Level

Beginner

Overview

Physical Problem Description

This example concerns the in-plane traction-comparison problem of an embedded plate subjected to two concentrated loads, as shown in the following diagram. This example illustrates the role of the different shell element formulations with regard to the mesh.

Units: mm, ms, g, N, MPa

The material used follows a linear elastic behavior and has the following characteristics:

•	Initial density: 7.8 x 10-3 g/mm3

•	Young modulus: 210000 MPa

•	Poisson ratio: 0.3

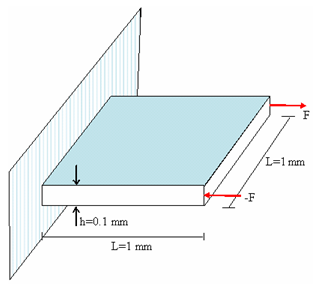
rad_ex_fig_18-9

Fig 9: Geometry of the problem.

Analysis, Assumptions and Modeling Description

Modeling Methodology

Four different types of mesh are used:

•	Mesh 1: two quadrilateral shells and four triangular shells (2Q4-4T3)

•	Mesh 2: four quadrilateral shells (4Q4)

•	Mesh 3: eight triangular shells (8T3)

•	Mesh 4: eight triangular shells (8T3 inverse)

For each model, the following shell formulations are tested:

•	QBAT formulation (Ishell =12)

•	QEPH formulation (Ishell =24)

•	Belytshcko & Tsay formulation (Ishell =1 or 3, hourglass control type 1, 3)

•	C0 and DKT18 formulations

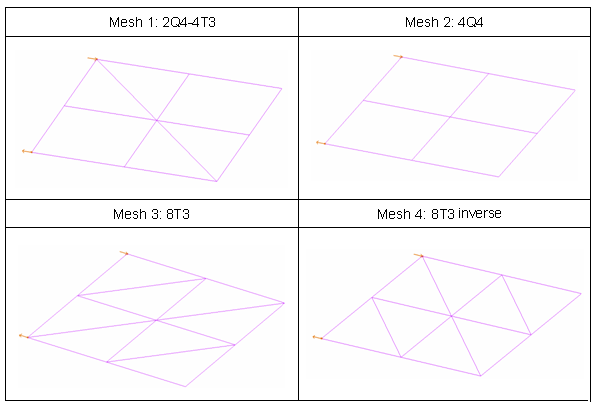
rad_ex_fig_18-18

Fig 10: Square plate meshes.

RADIOSS Options Used

The boundary conditions are such that the three nodes of a single side and the two middle ones are blocked, whereas the others are free with respect to the Y axis.

Two concentrated loads are applied on the corner points on opposing sides. They increase over time, as defined by the following function:

F(t)	0	10	10
t	0	200	400

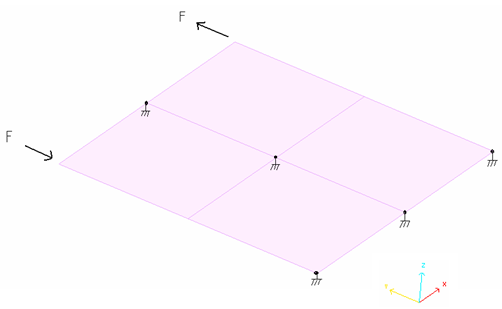
rad_ex_fig_18-11

Fig 11: Boundary conditions and loads.

Simulation Results

Curves and Animations

This example compares several models concerning:

•	the use of different element formulations for each mesh

•	the different types of mesh for a given element formulation

Two criteria used to compare the results are:

•	absorbed energy (internal and hourglass)

•	vertical displacement of the node under the loading point

The following diagrams summarize the results obtained.

Energy Curves / Comparison for Element Formulations

Mesh 1: 2Q4-4T3

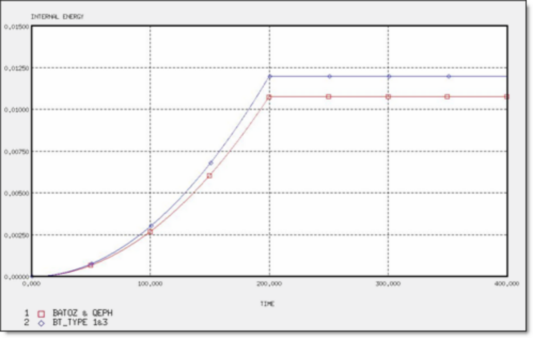
rad_ex_fig_18-12

Fig 12: Internal energy for 2 x Q4 and 4 x T3 elements.

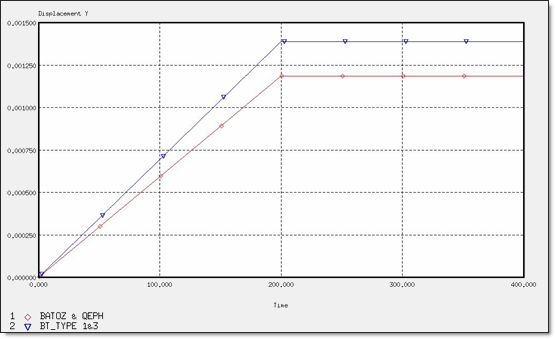
rad_ex_fig_18-13

Fig 13: Y Displacement for 2 x Q4 and 4 x T3 elements

Mesh 2: 4Q4

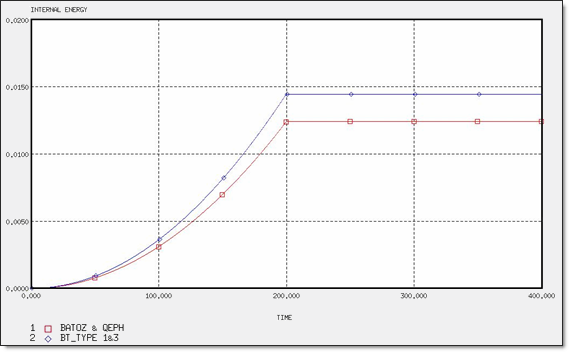
rad_ex_fig_18-14

Fig 14: Internal energy for 4 x Q4 elements.

Energy Curves / Comparison for Mesh Definitions

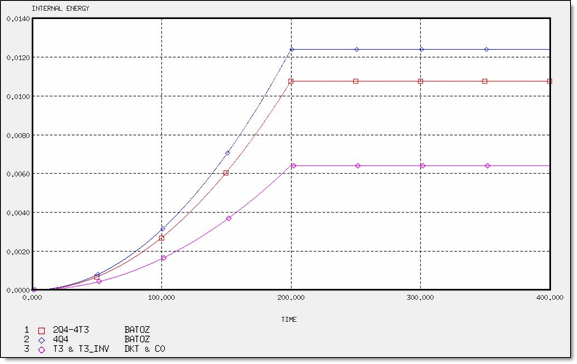
rad_ex_fig_18-15

Fig 15: Internal energy for different meshes.

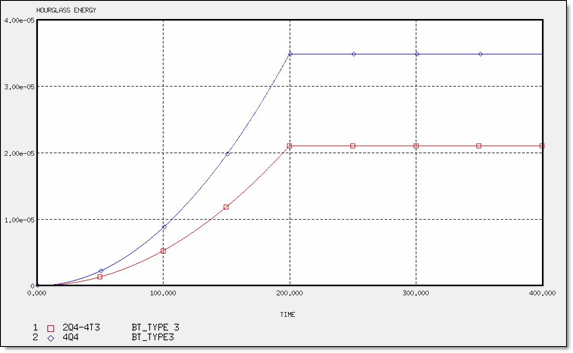
rad_ex_fig_18-16

Fig 16: Hourglass energy for different meshes.

Displacement and Maximum Energy Comparison

Elastic Plate	2Q4-4T3			4Q4			8T3		8T3_INV
Elastic Plate	QEPH	BT_TYPE 1 and 3	BATOZ	QEPH	BT_TYPE 1 and 3	BATOZ	DKT	CO	DKT	CO
IEmax	1.07 x 10-2	1.19 x 10-2	1.07 x 10-2	1.24 x 10-2	1.44 x 10-2	1.24 x 10-2	6.42 x 10-3	6.42 x 10-3	6.42 x 10-3	6.42 x 10-3
HEmax	---	2.10 x 10-5	---	---	3.49 x 10-6	---	---	---	---	---
Dymax	1.18 x 10-3 (Traction)	1.38 x 10-3 (Traction)	1.18 x 10-3 (Traction)	1.24 x 10-3	1.44 x 10-3	1.24 x 10-3	6.42 x 10-3	6.42 x 10-3	6.42 x 10-3	6.42 x 10-3

Conclusion

In the case of elastic flat plate modeling, when the loading is in-plane, the shell elements are reduced to become a membrane if the loads applied do not cause buckling.

A general overview of the results obtained highlight the following key points:

1.	The quadrilateral shell elements QEPH and QBAT have the same in-plane behavior.

2.	The different types of hourglass formulations in the BT shell elements lead to the same results, as there is no out-of-plane deformation and the material is supposed to be elastic.

3.	The three in-plane behaviors of the DKT18 and T3C0 RADIOSS triangles are exactly the same, as both of the elements are used for the same membrane formulation.

4.	The triangles are stiffer than the quadrilateral elements and do not provide good results, especially when the mesh is coarse.

Refer to the RADIOSS Theory Manual for more details.